ref : https://www.facebook.com/bhumisiam/posts/1306466159399506
สวัสดีครับแฟนเพจที่รักทุกๆ คน
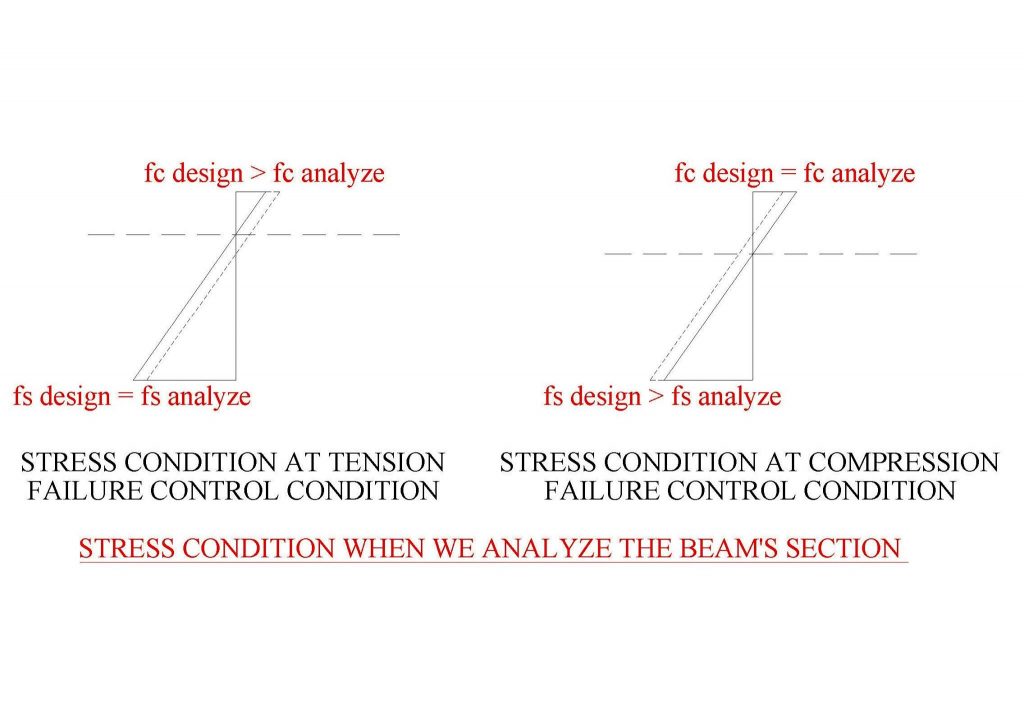
วันนี้ผมจะมาต่อถึงเนื้อหาที่ยังค้างทุกๆ คนนะครับ นั่นก็คือ เหตุใดทฤษฎีพื้นฐานของการออกแบบหน้าตัดคาน คสล ด้วยวิธีหน่วยแรงใช้งานจึงมีความสำคัญนะครับ ก่อนอื่นผมจะขอกล่าวถึงทฤษฎีกับอีก 1 สมการที่มีความสำคัญสมการหนึ่งก่อนครับ ขอให้ดูรูปที่ 1 ซึ่งจะแสดงให้เห็นถึงสภาวะความเค้นเมื่อเราทำการวิเคราะห์หน้าตัดคาน คสล ประกอบนะครับ เรื่องนั้นก็คือ เรื่องการกำหนดสภาวะการวิบัติของหน้าตัดคาน คือ หากเราเสริมเหล็กให้มีปริมาณน้อยกว่าค่าที่คำนวณได้จากใน BALANCE CONDITION การวิบัติจะเกิดขึ้นโดยถูกควบคุมด้วยแรงดึงเป็นหลัก (TENSION FAILURE CONTROL) ดังนั้นในสภาวะนี้ค่า fs ที่ใช้ในการวิเคราะห์จะมีค่าเท่ากับ fs ที่ใช้ในการออกแบบ แต่ ค่า fc ที่ใช้ในการวิเคราะห์จะมีค่าน้อยกว่า fc ที่ใช้ในการออกแบบ หรือ fs analyze = fs design แต่ fc analyze < fc design
ในทางตรงข้ามหากเราเสริมเหล็กให้มีปริมาณมากกว่าค่าที่คำนวณได้จากใน BALANCE CONDITION การวิบัติจะเกิดขึ้นโดยถูกควบคุมด้วยแรงอัดเป็นหลัก (COMPRESSION FAILURE CONTROL) ดังนั้นในสภาวะนี้ค่า fs ที่ใช้ในการวิเคราะห์จะมีค่าน้อยกว่า fs ที่ใช้ในการออกแบบ แต่ ค่า fc ที่ใช้ในการวิเคราะห์จะมีค่าเท่ากันกับ fc ที่ใช้ในการออกแบบ หรือ fs analyze < fs design แต่ fc analyze = fc design ด้วยเหตุประการฉะนี้เองคือจุดด้อยของวิธีการออกแบบหน้าตัดคาน คสล ด้วยวิธีหน่วยแรงใช้งาน คือ เมื่อทำการออกแบบหน้าตัดเราจะใช้ค่า fc และ fs เท่ากับค่าๆ หนึ่ง ทั้งๆ ที่ในความเป็นจริงแล้วไม่ใช่ กล่าวคือในการวิเคราะห์หน้าตัดจริง 1 หน้าตัดนั้นเราไม่สามารถที่จะกำหนดให้ค่าหน่วยแรงทั้งสองนี้มีค่าที่เท่ากันกับตอนที่ทำการออกแบบได้นั่นเองครับ
โดยจากก่อนหน้านี้เราทราบว่าค่าแรงดึง T และแรงอัด C จะมีค่าเท่ากับ
T = As fs
C = 1/2 fc k b d
ดังนั้นการจะทำให้หน้าตัดมีความสมดุลเราจะทราบว่าค่าทั้งสองจะต้องมีขนาดเท่ากัน
T = C
As fs = 1/2 fc k b d
As fs / fc = k b d / 2
โดยหากเราแทนค่าให้ p = As / bd ดังนั้น As = p b d
(p b d) fs / fc = k b d / 2
fs/fc = k / 2p
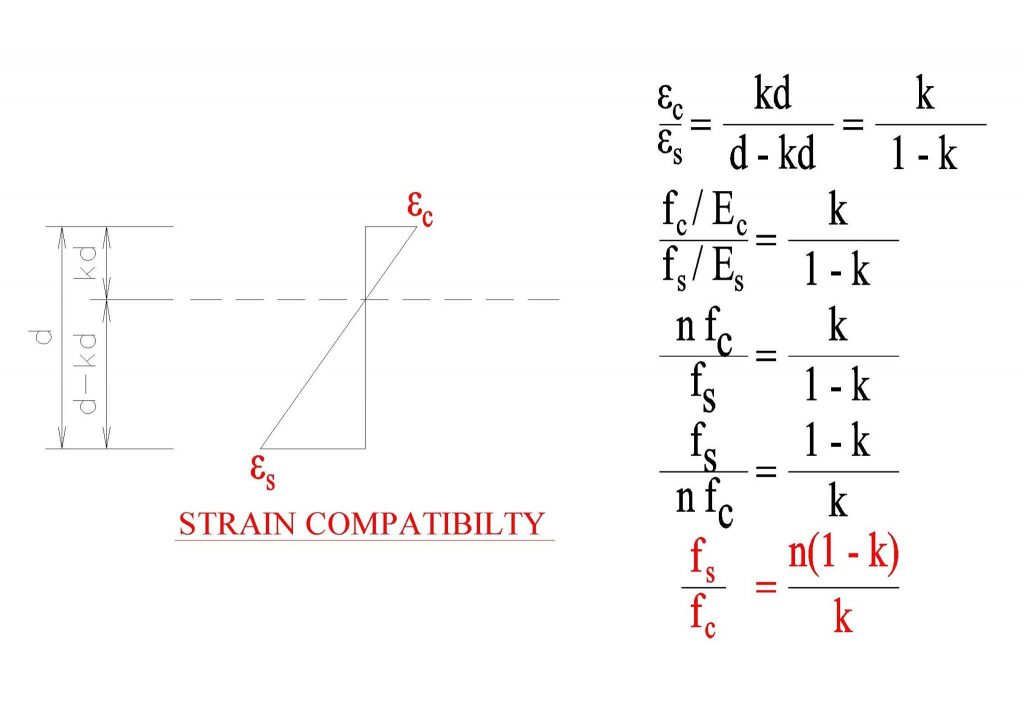
โดยจากในรูปที่ 2 หากเราแทนค่า fs / fc = n(1-k) / k
n(1-k) / k = k / 2p
เราสามารถแก้สมการหาค่า k ที่แท้จริงของหน้าตัดได้ว่า
k = SQRT[ 2np + (np)^(2) ] – np
เรามาดู ตย สั้นๆ กันสักข้อนะครับ
มีคานสี่เหลี่ยมผืนผ้าขนาดความกว้างเท่ากับ 20 cm ความลึกทั้งหมดเท่ากับ 50 cm ความลึกประสิทธผลของหน้าตัดเท่ากับ 45 cm กำหนดให้ใช้ค่า fc’ เท่ากับ 210 ksc ค่า fc เท่ากับ 65 ksc และค่า fs เท่ากับ 1,500 ksc โดยในหน้าตัดคานนี้จะได้รับการเสริมด้วยเหล็ก 3DB12mm เราจะมาลองหาคำตอบดูนะครับ
ก่อนอื่นเรามาเริ่มต้นจากสมการในการออกแบบก่อนนะครับ
Es = 2.04×10^(6) ksc
Ec = 15100SQRT(210) = 218820 ksc
n = 2.04×10^(6)/218,820 = 9.32 ~ 10
As = (3)(1.13) = 3.39 cm^(2)
p = 3.39/(20×45) = 0.0038
np = 0.038
k = 1/(1+1,500/10×65) = 0.302
j = 1 – 0.302/3 = 0.899
Mc = (1/2)(65)(0.899)(0.302)(20)(45)^(2)/100,000 = 3.5 T-m
Ms = (3.39)(1,500)(0.899)(45)/100,000 = 2 T-m
ดังนั้นคานนี้มีการเสริมเหล็กเป็นแบบ TENSION FAILURE DESIGN ดังนั้นในการวิเคราะห์หน้าตัดค่าหน่วยแรง fc ที่จะใช้ในการแทนค่าจะมีค่าน้อยกว่า fc ที่ใช้ในการออกแบบ ดังนั้นหากทำการแทนค่าจะพบว่า
k = SQRT[ (2)(0.038) + (0.038)^(2) ] – (0.038) = 0.239
จะเห็นได้ว่าค่า k ในสภาวะของการวิเคราะห์หน้าตัด (k=0.239) จะมีค่าน้อยกว่าค่า k ที่ใช้ในการออกแบบซึ่งจะสอดคล้องกับการกำหนดลักษณะรูปแบบของการวิบัติในคานของเรา (k=0.302)
ทำการแก้สมการค่าอื่นๆ จะได้ว่า
j = 0.92
fc = 48 ksc
Mc = (1/2)(48)(0.92)(0.239)(20)(45)^(2)/100,000 = 2.1 T-m
Ms = (3.39)(1,500)(0.92)(45)/100,000 = 2.1 T-m
จะเห็นได้ว่าหากอาศัยการหาค่า k ตามทฤษฎีข้างต้น ค่าโมเมนต์ที่รับได้โดยทั้งคอนกรีตและเหล็กเสริมจะได้ค่าที่ตรงกัน ดังนั้นหากเพื่อนๆ ไม่เข้าใจถึงหลักการและสมการพื้นฐานเหล่านี้ ก็อาจจะกลายเป็นความคุ้นเคยที่ว่าค่าโมเมนต์ที่คานจะสามารถรับได้โดยการวิเคราะห์หน้าตัดจริงจะมีค่าเท่ากันกับที่เราได้ทำเอาไว้ในขั้นตอนการออกแบบ ซึ่งไม่ถูกต้องนั่นเองครับ โดยหากเราอาศัยวิธีการออกแบบโดยวิธีกำลังเราจะไม่ประสบและพบเจอปัญหาในลักษณะแบบนี้นะครับ นี้เองเป็นส่วนหนึ่งที่ว่าเหตุใดจึงมีคำกล่าวที่ว่าวิธีการออกแบบโครงสร้าง คสล ด้วยวิธีกำลังจึงมีความเหมาะสมกว่าวิธีหน่วยแรงใช้งาน
Today I shall continue our lesson from yesterday about why the basis theory of reinforced concrete beam design using the working stress design method is quite important.
Before I continue explaining I shall first give you one more important theory and equation. See the attached figure 1 showing the stage when we analyze the section. The theory is about the failure stage of the beam’s section.
If we reinforced the section under the balance condition the beam will now be in a tension control mode.
Hence, the stress fs in the section analysis will be equal to the design fs, but the stress fc will be less than the design fc or:
fs analyze = fs design แต่ fc analyze < fc design
In the other hand, if we reinforced the section over the balance condition the beam will now be in a compression control mode.
Hence, the stress fs in the section analysis will be less than the design fs, but the stress fc will now be equal to the design fc or:
fs analyze < fs design แต่ fc analyze = fc design
This is one of the disadvantages of using the working stress design method. That is when we design a value of moment we first obtain one parameter, but when we analyze the section using the same parameter we now receive a different result of moment capacity from the same section of beam.
Before this we already knew that the T and C will be equal to:
T = As fs
C = 1/2 fc k b d
To make the section equilibrium we must equalize these two forces. Thus;
T = C
As fs = 1/2 fc k b d
As fs / fc = k b d / 2
Substitute p = As / bd, hence As = p b d
(p b d) fs / fc = k b d / 2
fs/fc = k / 2p
From the attached figure 2 we now substitute fs / fc = n(1-k) / k
n(1-k) / k = k / 2p
We can now compute the actual k of the section equal to:
k = SQRT[ 2np + (np)^(2) ] – np
Let’s see a short example for this problem
A rectangular beam consisting of width equal to 20 cm. The total depth is 50 cm, and the effective depth is equal to 45 cm. Given fc’ equal to 210 ksc, and fc equal to 65 ksc, and fs will be equal to 1,500 ksc. The beam is reinforced by a 3 no. of DB12mm reinforced steel. Let’s compute this together.
First compute the design equations:
Es = 2.04×10^(6) ksc
Ec = 15100SQRT(210) = 218820 ksc
n = 2.04×10^(6)/218,820 = 9.32 ~ 10
As = (3)(1.13) = 3.39 cm^(2)
p = 3.39/(20×45) = 0.0038
np = 0.038
k = 1/(1+1,500/10×65) = 0.302
j = 1 – 0.302/3 = 0.899
Mc = (1/2)(65)(0.899)(0.302)(20)(45)^(2)/100,000 = 3.5 T-m
Ms = (3.39)(1,500)(0.899)(45)/100,000 = 2 T-m
Hence, the beam will be in a tension failure design mode, so the stress fc in the analysis will be less than the design fc. If we substitute all the values we now found that:
k = SQRT[ (2)(0.038) + (0.038)^(2) ] – (0.038) = 0.239
We can now see that the analyze k value (k=0.239) will be smaller than the design k which this will be according to the theory of failure mode introduced earlier (k=0.302)
Solve other equations now yields:
j = 0.92
fc = 48 ksc
Mc = (1/2)(48)(0.92)(0.239)(20)(45)^(2)/100,000 = 2.1 T-m
Ms = (3.39)(1,500)(0.92)(45)/100,000 = 2.1 T-m
We can investigate that if we obtain the value of k from the purposed theory the moment capacity contribute from the concrete and steel will be similar with each other. So if you guys just don’t understand these theory and basic equations it might cause you to misunderstood that the moment capacity from the analyze section will be similar to the design process which is incorrect. Thus, in applying the strength method we will not met these type of problems. This is why we often heard that the strength design method is a better option in designing an RC section more than the working stress design method.
ADMIN JAMES DEAN
BSP-Bhumisiam
เสาเข็ม สปันไมโครไพล์ ช่วยแก้ปัญหาได้เพราะ
1) สามารถทำงานในที่แคบได้
2) ไม่ก่อให้เกิดมลภาวะทางเสียง
3) หน้างานสะอาด ไม่มีดินโคลน
4) สามารถรับน้ำหนักได้ 20-40 ตัน/ต้น
5) สามารถตอกชิดผนังกำแพง ไม่ทำให้โครงสร้างเดิมเสียหาย
สนใจติดต่อสินค้า เสาเข็ม ไมโครไพล์ (Micropile) สปันไมโครไพล์ (Spun MicroPile) มาตรฐาน มอก. ของ ภูมิสยาม ซัพพลาย ติดต่อ สายด่วน โทร 081-634-6586
http://www.micro-pile.com